Applications: Area Between Curves III

Problem 246: Find the area of the region that is enclosed between $y = 7x^2$ and $y = x^2 + 6$ .

Solution: In order to find the area enclosed by the two functions, we need to find the limits of integration. That is, since we have two functions equal to $y$ , we can make it equal to each other.

(1) $\begin{equation*} 7x^2 = x^2 + 6 \implies 7x^2-x^2-6 = 0 \implies x^3 - x =0 \implies x = 0, \pm 1.\end{equation*}$

Let us now find the area of the region. A graph is always helpful when doing these type of problems.

(2) $\begin{align*} A & = \int_{a}^{b} \text{Top/Right Function-Bottom/Left Function} \ dx \\ & = \int_{-1}^{1} x^2 + 6 - 7x^2 \ dx \\ & = \int_{-1}^{1} 6 - 6x^2 \ dx \\ & = 6x -\frac{6}{3}x^3\bigg|_{-1}^{1} \\ & = 8 . \end{align*}$

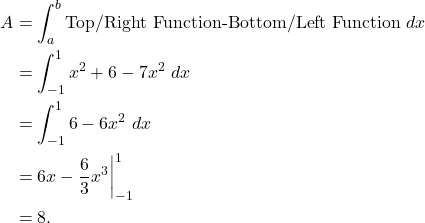
That is, the area of the region enclosed between the two given functions is 8.

One Problem at a Time

Applications: Area Between Curves III

Leave a Reply Cancel reply