Problem 200: Solve the system by using substitution 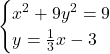 .
.
Solution: Using equation two and plugging it into equation one we have
(1) 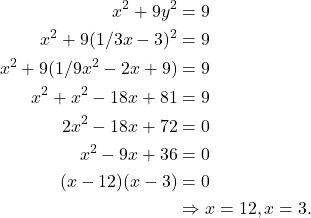
Using this ![]() ‘s values we can find the
‘s values we can find the ![]() ‘s values. That is,
‘s values. That is,
![]() :
:
(2) ![]()
![]() :
:
(3) ![]()
Thus the potential solutions are ![]() and
and ![]() . However, we need to check that this potential solutions satisfy the two equations. Hence,
. However, we need to check that this potential solutions satisfy the two equations. Hence,
![]() :
:
![Rendered by QuickLaTeX.com \[\begin{cases} x^2 + 9y^2 = 9 \quad \Rightarrow \quad (12)^2 + 9(1)^2 \ne 9 \\ y = \frac{1}{3}x - 3 \quad \Rightarrow \quad 1 = \frac{1}{3}(12) - 3 \end{cases}\]](https://1problematatime.com/wp-content/ql-cache/quicklatex.com-6fa8b81d389235941dc4b6785b36384e_l3.png)
Since the point ![]() does not satisfies both equations, this point is not a solution of the system.
does not satisfies both equations, this point is not a solution of the system.
![]() :
:
![Rendered by QuickLaTeX.com \[\begin{cases} x^2 + 9y^2 = 9 \quad \Rightarrow \quad (3)^2 + 9(-2)^2 \ne 9 \\ y = \frac{1}{3}x - 3 \quad \Rightarrow \quad -2 = \frac{1}{3}(3) - 3 \end{cases}\]](https://1problematatime.com/wp-content/ql-cache/quicklatex.com-60e6199741bfcd647054412875bb0aa5_l3.png)
Since the point ![]() does not satisfies both equations, this point is not a solution of the system.
does not satisfies both equations, this point is not a solution of the system.
Therefore, the system has no solutions.

Leave a Reply