Application of Area

Problem 192: A rectangular bedroom has an area of 117 square root. The length of the bedroom is four feet more than the width. Find the length and width.

Solution: We know that the area of a rectangle is given by

(1) $\begin{equation*} A = l \cdot w, \end{equation*}$

where $l$ is the length of the rectangle and $w$ is the width. Since the length of the bedroom is four feet more than the width, we have $l = w + 4$ . That is,

(2) $\begin{align*} A & = l \cdot w \\ 117 & = (w + 4) \cdot w \\ 117 & = w^2 + 4w \\ 0 & = w^2 + 4w - 117 \\ 0 & = (w+13)(w-9) \\ & \Rightarrow w =-13, w = 9. \\ \end{align*}$

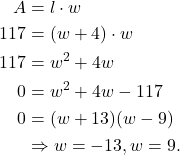
Since the width can’t be negative, we have $w = 9$ . Hence, $l = w + 4 = 9 + 4 = 13$ .

Therefore, the rectangle has length of 13 feet and width of 9 feet.

Leave a Reply Cancel reply