Cost from Marginal Cost

Problem 189: The marginal cost of printing a poster when $x$ posters have been printed is

(1) $\begin{equation*} \frac{dc}{dx} = \frac{1}{2\sqrt{x}}\end{equation*}$

dollars. Find $c(100)-c(1)$ , the cost of printing posters $2-100$ .

Solution: First, we need to find our function $c(x)$ , and then find the cost of printing posters $2-100$ . That is,

(2) $\begin{align*} \frac{dc}{dx} & = \frac{1}{2\sqrt{x}} \\ dc & = \frac{1}{2\sqrt{x}}dx \\ \int_{1}^{100} dc & = \int_{1}^{100} \frac{1}{2\sqrt{x}} dx \\ c(x) & = \sqrt{x}\bigg|_{1}^{100} \\ & = \sqrt{100} - \sqrt{1} \\ & = 10 - 1=9. \end{align*}$

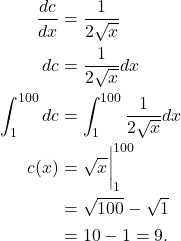
Hence, the cost of printing posters $2 -100$ is $9.00.

Leave a Reply Cancel reply