Problem 188: Find ![]() if
if
(1) ![]()
Solution: Using the Fundamental Theorem of Calculus (Part 1), we have
(2) 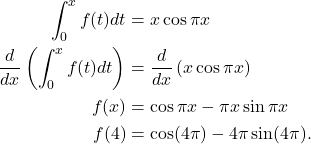
Hence,
(3) ![]()

Problem 188: Find ![]() if
if
(1) ![]()
Solution: Using the Fundamental Theorem of Calculus (Part 1), we have
(2) 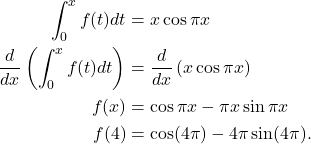
Hence,
(3) ![]()
Leave a Reply