Problem 185: Find the linearization of
(1) ![]()
at ![]() .
.
Solution: The linearization at a point is given by
(2) ![]()
where ![]() is the point
is the point ![]() . Then
. Then
(3) 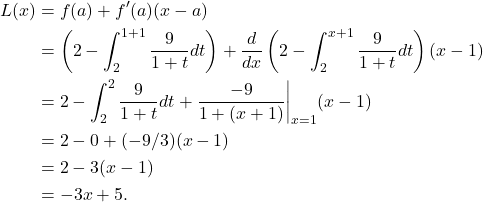
Hence , at ![]() , the linearization is given by
, the linearization is given by
(4) ![]()

Problem 185: Find the linearization of
(1) ![]()
at ![]() .
.
Solution: The linearization at a point is given by
(2) ![]()
where ![]() is the point
is the point ![]() . Then
. Then
(3) 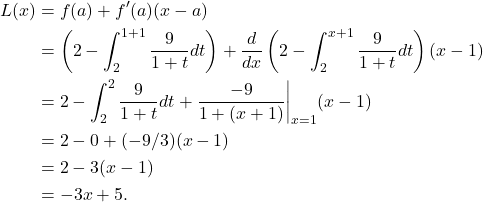
Hence , at ![]() , the linearization is given by
, the linearization is given by
(4) ![]()
Leave a Reply