Concavity of A Function

Problem 174: Determine the concavity of $y=3 + \sin x$ on $[0, 2\pi]$ .

Solution: In order to find the concavity of a differentiable function, we use the second derivative. That is,

(1) $\begin{align*} y(x) &=3 + \sin x \\ y'(x) & = \cos x \\ y"(x) & = -\sin x.\end{align*}$

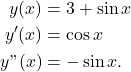
We have a change of concavity at the inflection point (that is, $y"(x)=0$ ). Then we have

$y"(x) = -\sin x = 0 \quad \Rightarrow \quad x = \pi, 2\pi.$

Since $f"(x)= - \sin x < 0$ on the interval $(0, \pi)$ , the function $f(x)$ is concave down on the interval $(0, \pi)$ .

Likewise, since $f"(x)= - \sin x > 0$ on the interval $(\pi, 2\pi)$ , the function $f(x)$ is concave up on the interval $(\pi, 2\pi)$ .