Problem 162: Show that ![]() , where
, where ![]() is a differentiable function and
is a differentiable function and ![]() is an arbitrary base.
is an arbitrary base.
Solution: Using the relationship between ![]() and
and ![]() ,
,
(1) ![]()
Then
(2) 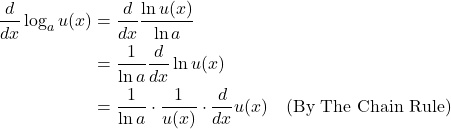
That is,
(3) ![]()

Problem 162: Show that ![]() , where
, where ![]() is a differentiable function and
is a differentiable function and ![]() is an arbitrary base.
is an arbitrary base.
Solution: Using the relationship between ![]() and
and ![]() ,
,
(1) ![]()
Then
(2) 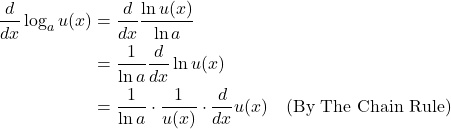
That is,
(3) ![]()
Leave a Reply