Problem 178: Find a formula for the Riemann sum obtained by dividing the interval ![]() into
into ![]() equal subintervals and using the right-hand endpoint for each
equal subintervals and using the right-hand endpoint for each ![]() . Then take a limit of these sums as
. Then take a limit of these sums as ![]() to calculate the area under the curve over
to calculate the area under the curve over ![]() .
.
![]()
Solution: We will divide the interval ![]() into
into ![]() subintervals. Since the subintervals have the same length, the length of the subintervals is given by
subintervals. Since the subintervals have the same length, the length of the subintervals is given by
![]()
The height of the subintervals is given by
![]()
which is the height of the function at each right-hand point of the subintervals. That is, the Riemann sum is given by
(1) 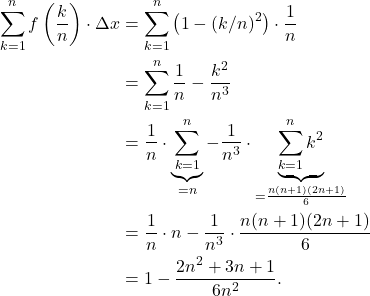
Taking the limit,
(2) 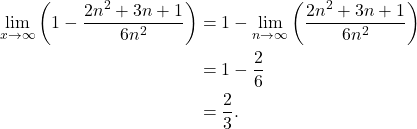
Hence, the area under the curve over the interval ![]() is
is ![]() .
.

Leave a Reply